To verify the mathematical model of ELPLA for rigid circular raft, results of a rigid circular raft obtained by other analytical solutions from Borowicka (1939) and Stark (1990) (Section 5.2, page 106) are compared with those obtained by ELPLA.
The vertical displacement w [m] of a rigid circular raft on Isotropic elastic-half space medium may be evaluated According to Borowicka (1939).
Example 9: Rigid circular raft on Isotropic elastic-half space medium
Description of the problem
To verify the mathematical model of ELPLA for analyzing continuous beams, results of a continuous beam introduced by Harry (1993) (Examples 10.2, 10.4 and 10.5, pages 399, 409 and 411) are compared with those obtained by ELPLA.
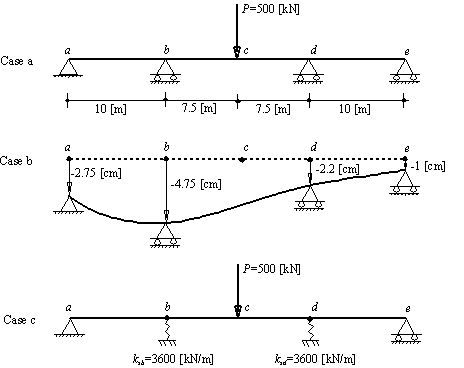
A continuous beam of length L = 35 [m] is chosen as shown in Figure 60. The beam is subjected to a point load of P = 500 [kN] at the center. The beam cross section yields Moment of Inertia I = 0.003 [m4]. Young's modulus of the beam is E = 2.0×108[kN/m2].
For the comparison, three different cases are considered as follows:
Case a: Continuous beam with a point load P at the center on supports at points a, b, d and e.
Case b: Instead of the point load P at the center of the beam, points a, b, d and e have the following support settlements: Δa = -2.75 [cm], Δb = -4.75 [cm], Δd = -2.2 [cm] and Δe = -1.0 [cm].
Case c: Points c and d are supported by elastic springs that have stiffness of ksb = ksd = 3600 [kN/m].
Description of the problem
To verify the consolidation settlement calculated by ELPLA, the final consolidation settlement of a clay layer under a circular footing calculated by Das (1983) (Example 6.3, page 371) is compared with that obtained by ELPLA.
A circular footing 2 [m] in diameter at a depth of 1.0 [m] below the ground surface is considered as shown in Figure 7. Water table located at 1.5 [m] below the ground surface. The contact pressure under the footing is assumed to be uniformly distributed and equal to q = 150 [kN/m2]. A normally consolidated clay layer 5 [m] thick is located at a depth of 2.0 [m] below the ground surface. The soil profile is shown in the Figure. It is required to determine the final settlement under the center of the footing due to consolidation of the clay.
Example 7: Consolidation settlement under a circular footing
Description of the problem
In many cases, it is required to determine the settlement under an abutment, a bridge pier, a building core or a raft of thick thickness. In these cases, the foundation will be assumed as rigid foundation.
As an example for rigid rafts, consider the rectangular raft of a core from concrete walls shown in Figure 55 as a part of 93.0 [m] structure. The length of the raft is L = 28.0 [m], while the width is B = 25.0 [m]. Due to the lateral applied wind pressure, the raft subjected to an eccentric vertical load of P = 142000 [kN]. Figure 55 shows section elevation through the raft and subsoil, while Figure 56 shows a plan of the raft, load, dimensions and mesh.
It is required to estimate the expected settlement if the raft is considered as perfectly rigid.
Example 23: Settlement calculation for a rigid raft subjected to an eccentric load
Description of the problem
To verify the mathematical model of ELPLA for analyzing grid foundations, the results of grid foundation on elastic springs obtained by Szilard (1986) (Example 4.4.5, page 350) are compared with those obtained by ELPLA.
The geometry and the loads of foundation are the same as those of Szilard (1986) as shown in Figure 21. The grid has rectangular cross section of 2.5 [m] width and 0.5 [m] depth, yields Moment of Inertia I = 0.026 [m4] and Torsion modulus J = 0.091 [m4]. The parameters of grid material are Young's modulus Eb = 3×10 [kN/m] and Shear modulus Gb = 1×10 [kN/m]. Modulus of subgrade reaction of the soil is ks = 40000 [kN/m].
GEOTEC Software is providing universities and consulting companies with the right tools for the last 25+ years. We also assist our clients in various geostructural projects. From basic to advanced problems, GEOTEC Software can provide you with the right technical assistant.
Select your language
